We've talked about the Doppler shift in several different contexts. One was the original transit system, NNSS system, that operated on the Doppler shift. As the satellite passes overhead, the range between the receiver and the satellite changes; that steady change is reflected in a smooth and continuous movement of the phase of the signal coming into the receiver. GPS uses the Doppler shift as an observable. It has broad applications in signal processing. It can be used to discriminate between the signals from various GPS satellites, to determine integer ambiguities in kinematic surveying, as a help in the detection of cycle slips, and as an additional independent observable for autonomous point positioning. But perhaps the most important application of Doppler data is the determination of the range rate between a receiver and a satellite. Range rate is a term used to mean the rate at which the range between a satellite and a receiver changes over a particular period of time.
Typical Change in the Doppler Shift
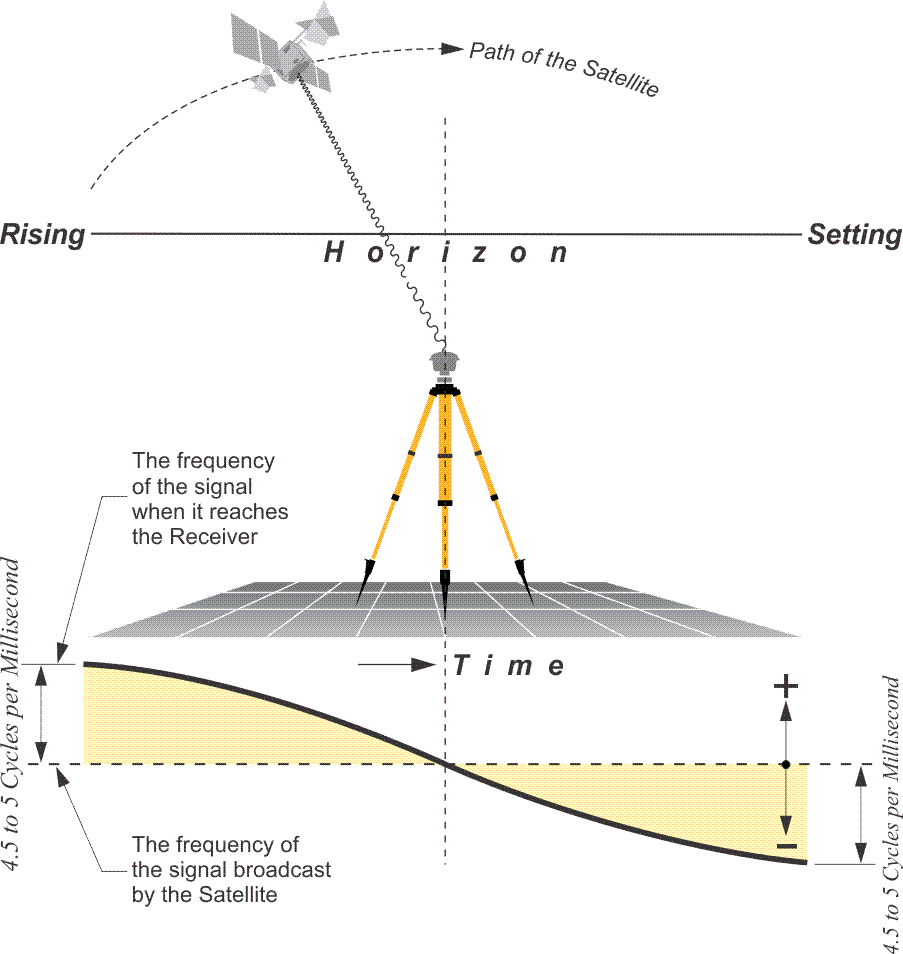
This graphic shows the typical rate of change in the Doppler shift with regard to a stationary, static, GPS receiver. The signal received would have its maximum Doppler shift, +4 1/2 to 5 cycles per millisecond, when the satellite is at its maximum range, just as it is rising or setting. The Doppler shift continuously changes throughout the overhead pass. Immediately after the satellite rises, relative to a particular receiver, its Doppler shift gets smaller and smaller, until the satellite reaches its closest approach, at zenith. At that moment its radial velocity with respect to the receiver is zero, the Doppler shift of the signal is zero as well. But as the satellite recedes, it grows again, negatively, until the Doppler shift once again reaches its maximum extent just as the satellite sets, -4 1/2 to 5 cycles per millisecond. It is very predictable. That predictability, the constant variation of the signal's Doppler shift, makes it a good observable. If the receiver's oscillator frequency is adjusted to match these variations exactly, as they're happening, it will duplicate the incoming signal's shift and phase. This strategy of making measurements using the carrier beat phase observable is a matter of counting the elapsed cycles and adding the fractional phase of the receiver's own oscillator. This is one way that the phase lock loop maintains its lock on the signal as the Doppler shift occurs with each of the satellites that it is tracking.
With respect to the receiver, the satellite is always in motion even if the receiver is static. But the receiver may be in motion in another sense, as it is in kinematic GPS. The ability to determine the instantaneous velocity of a moving vehicle has always been a primary application of GPS, and is based on the fact that the Doppler-shift frequency of a satellite’s signal is nearly proportional to its range rate. The Doppler shift can be used to discriminate between the signals of the various satellites to help in the determination of the integer ambiguity. It can help in detection of a loss of lock due to a cycle slip.
Continuously Integrated Doppler
The Doppler-shift and the carrier phase are measured by first combining the received frequencies with the nominally constant reference frequency created by the receiver’s oscillator. The difference between the two is the often mentioned beat frequency, an intermediate frequency, and the number of beats over a given time interval is known as the Doppler count for that interval. Since the beats can be counted much more precisely than their continuously changing frequency can be measured, most GPS receivers just keep track of the accumulated cycles, the Doppler count. The sum of consecutive Doppler counts from an entire satellite pass is often stored, and the data can then be treated like a sequential series of biased range differences. Continuously Integrated Doppler is such a process. The rate of the change in the continuously integrated Doppler shift of the incoming signal is the same as that of the reconstructed carrier phase. Integration of the Doppler frequency offset results in an accurate measurement of the advance in carrier phase between epochs. And as stated earlier, using double-differences in processing the carrier phase observables removes most of the error sources other than multipath and receiver noise.