Trends, Weather, and Climate Change
On June 29, 2021, the mercury at Portland, Oregon reached 116oF. December 10 and 11, 2021 saw EF4 tornadoes roar through Kentucky and other states, killing 88 and wounding more than 630. In December 2021, 202 inches of snow fell in the Sierra Nevada of California. Hurricane Sandy made landfall in New Jersey on October 29th, 2012, a very late point in the year for a storm to reach the northeastern US coast. January 8th, 2013 was the hottest day ever for Australia with an average temperature for the entire continent of 40.3oC (nearly 105oF). The AVERAGE (day and night) temperature in Phoenix in June 2017 was almost 95 degrees. And nearly 61 inches of rain fell around Houston during Hurricane Harvey in August 2017. Each of these events provoked arguments in favor or against global warming. Yet, on their own, none of them were definitive proof one way or another.
To make a case for climate change, we need to find the average global climate signal, which is often difficult to discern from the noise of short-term variations and regional differences associated with what we call weather. It is very important to remember that climate is the time-averaged weather, so when we are talking about climate change, we are not talking about the weather that you experience on a daily or seasonal basis — a single heat wave is not evidence of global climate warming, just as one cold snap does not constitute global climate cooling. But repeated, unusual heat waves will shift the average temperature of a region, and this can be taken as a manifestation of a warming climate. The climate is inherently variable over time and space, so detecting a meaningful trend is a challenge that requires great care, a lot of data and often some complex statistics.
Now, a word of warning, you might find the remainder of this page a little dull! However, it happens to be one of the most essential topics of the whole issue of climate change. We absolutely have to understand the significance of trends if we are going to interpret them!
Let’s consider a hypothetical case to help you better understand the nature of the problem. The mean annual temperature is the average temperature over the course of a year, and it varies in a way that we can simulate with a randomly generated string of numbers (geoscientists often refer to such variation as “noise”) added to a constant, long-term mean temperature. We would see something like this:
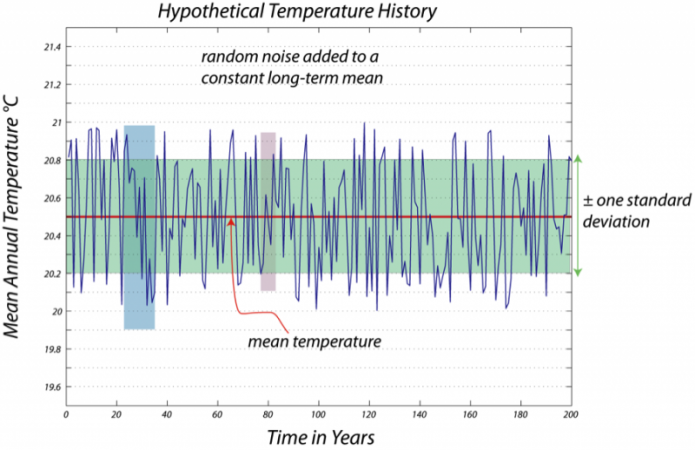
In this case, you might say that if the temperature strayed out of the green zone, you have unusually hot or cold weather, and you would expect this kind of temperature excursion to be a standard feature of the natural variability of the region's climate. But, the green zone has more or less fixed limits, so the standard for calling something a heat wave does not change over time.
Now, let’s look at another hypothetical case where the climate really is warming, but there is still the natural variability or noise on a shorter timescale.

If you think of the upper edge of the green zone on the previous figure as indicating the line for defining a heat wave, look what happens in a case where there is a steady warming of the climate, with the same kind of weather causing the rapid ups and downs. If at time 0, we said that a temperature of 21°C was a heat wave, that becomes the mean climate temperature by time 60 in the above figure — so, what previously was a rare warm spell is now just the standard. This means that, by older standards, "heat waves" become more common as time goes on.
Note that, once again, we can find areas in this above figure where a shorter time period would seem to indicate cooling or warming. This reinforces the idea that we can’t really talk about climate change by looking at just a few years, and leads to the question of how much time we do need to look at to get a good understanding of climate change. In general, the longer, the better. But just to illustrate this, consider the following, where we take the same kind of hypothetical temperature record as above and systematically find the linear trends over time, with windows of varying length that slide along through the 200-year record.
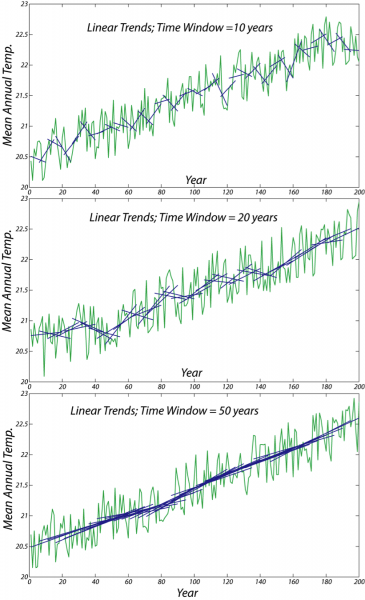
Why do we care about this? There are all sorts of reasons why this is important and interesting, but here are three primary reasons.
- The first reason for studying recent climate is that if we understand how climate has been changing in the recent past, we can establish a trend that we can use to project into the future.
- The second reason for studying recent climate is that knowing the history of climate change gives us a chance to understand how the climate system responds to various controls; for instance, we know the histories of solar insolation, fossil fuel burning, land clearing, addition of other pollutants to the atmosphere (other greenhouse gases and particles that block sunlight), and volcanic eruptions. Knowing these histories and knowing how the climate has changed, we are in a position to develop a good understanding of the role that each of these controls plays in changing the climate, which helps us predict future climate change with greater confidence.
- Finally, if climate change is real, then we cannot ignore it when planning for our future. The consequences of future climate change may require some difficult choices, so we had better be sure that there is a firm basis for the reality of climate change.
